Horizontal Tangent Line Implicit Differentiation
Andymath.com features costless videos, notes, and practice problems with answers! Printable pages brand math like shooting fish in a barrel. Are you ready to be a mathmagician?
Notes
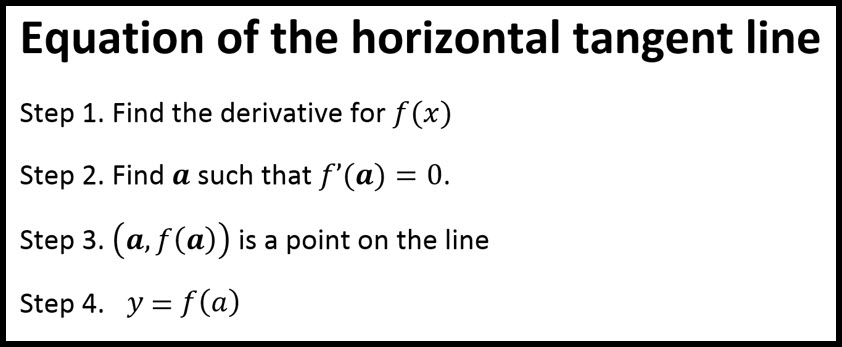
Questions
Find the equations of the horizontal tangent lines.
\(\textbf{1)}\) \( f(x)=10^two+4x+4 \)
The answer is \( y=0 \)
\(\textbf{ii)}\) \( f(ten)=\sin 10 \)
The respond is \( y=1 \) and \( y=-ane \)
\(\textbf{3)}\) \( f(x)=4 \)
The respond is \( y=4 \)
Come across Related Pages\(\)
\(\bullet\text{ Definition of Derivative}\)
\(\,\,\,\,\,\,\,\, \displaystyle \lim_{\Delta x\to 0} \frac{f(x+ \Delta ten)-f(x)}{\Delta x} \)
\(\bullet\text{ Equation of the Tangent Line}\)
\(\,\,\,\,\,\,\,\,f(ten)=x^3+3x^2−ten \text{ at the bespeak } (two,eighteen)\)
\(\bullet\text{ Derivatives- Constant Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(c)=0\)
\(\bullet\text{ Derivatives- Power Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(x^n)=nx^{n-1}\)
\(\bullet\text{ Derivatives- Constant Multiple Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}(cf(x))=cf'(x)\)
\(\bullet\text{ Derivatives- Sum and Difference Rules}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(x) \pm g(ten)]=f'(ten) \pm thousand'(x)\)
\(\bullet\text{ Derivatives- Sin and Cos}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}sin(10)=cos(x)\)
\(\bullet\text{ Derivatives- Product Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(ten) \cdot g(ten)]=f(x) \cdot chiliad'(ten)+f'(ten) \cdot 1000(x)\)
\(\bullet\text{ Derivatives- Quotient Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}\left[\displaystyle\frac{f(x)}{g(10)}\right]=\displaystyle\frac{g(x) \cdot f'(x)-f(x) \cdot g'(x)}{[g(10)]^2}\)
\(\bullet\text{ Derivatives- Concatenation Rule}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[f(g(10))]= f'(g(x)) \cdot g'(x)\)
\(\bullet\text{ Derivatives- ln(10)}\)
\(\,\,\,\,\,\,\,\,\displaystyle\frac{d}{dx}[ln(x)]= \displaystyle \frac{ane}{10}\)
\(\bullet\text{ Implicit Differentiation}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Horizontal Tangent Line}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Mean Value Theorem}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Related Rates}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Increasing and Decreasing Intervals}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Intervals of concave upward and downward}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Inflection Points}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Graph of f(x), f'(x) and f"(x)}\)
\(\,\,\,\,\,\,\,\,\)
\(\bullet\text{ Newton's Method}\)
\(\,\,\,\,\,\,\,\,x_{n+i}=x_n – \displaystyle \frac{f(x_n)}{f'(x_n)}\)
Search More Math Topics
\(\bullet\text{ Andymath Homepage}\)
Delight Subscribe on Youtube

Horizontal Tangent Line Implicit Differentiation,
Source: https://andymath.com/horizontal-tangent-line/
Posted by: jinksavenifelf.blogspot.com






0 Response to "Horizontal Tangent Line Implicit Differentiation"
Post a Comment